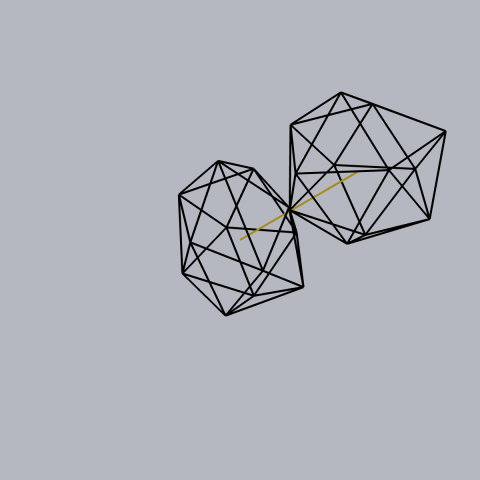
Creating the backbone of the nucleus

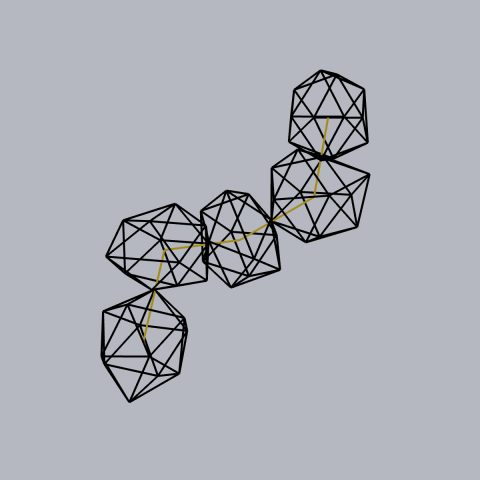
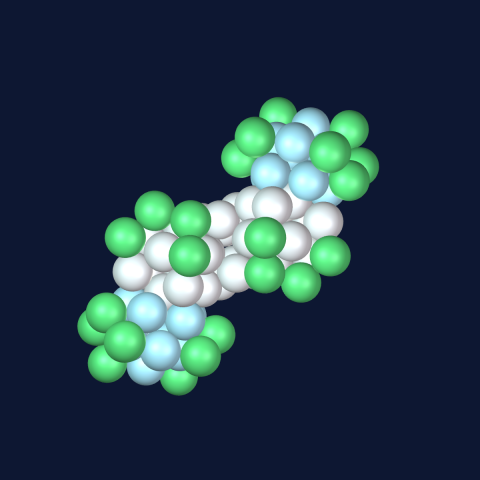
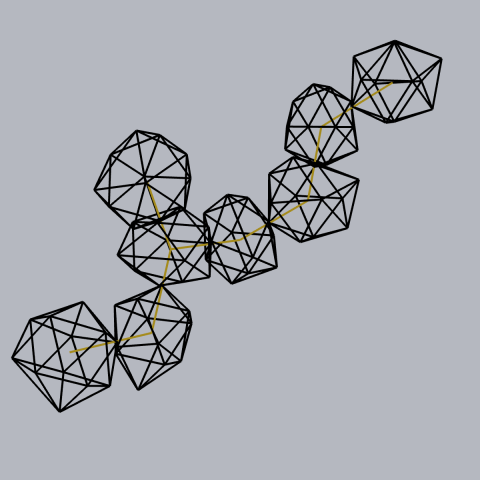
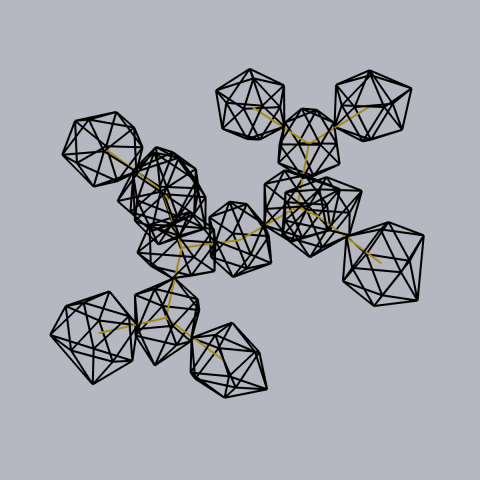
The growth process described so far results in a backbone of carbon nuclets that constitutes the bulk of the make-up of every nucleus. The element properties are determined by the various active endings, which also drive the outer electron structure, thus explaining the similarity of those elements belonging to the same element group. The backbone of icosahedrons is created in fractal fashion. It does this in two different ways: through branching and by elongation. We can also distinguish an active topside with visible endings and inert backside with more of the backbone visible.

The next several figures show how the icosahedrons are connected to each other in SAM for various groups of elements in the PTE. Branching and elongation of the core structures is the result of the initial distortion of the primary icosahedron.

In addition, we see branches start to interfere with each other, which introduces stress in the structure and is a fundamental cause of a nuclide being radioactive. In the overall growth process, we can distinguish four organizational patterns.

• First order = single nuclet. The first-order pattern reaches its limit at the completion of the ideal platonic solid (icosahedron configuration) which is the structure of carbon.
• Second order = two nuclets. This is the doubling factor that creates the fractal form of the larger elements. Growth on the two growth points must be balanced, otherwise the nucleus will not be stable. The last nucleus with only second-order organization is phosphorus.
• Third order = fractal growth on several growth points in parallel. This fractal growth in essence competes with the spherical densest packing rule. The addition of protons will follow the first and second order rules where possible. This is a balancing act between the branches, related to nuclear reactions, isotope stability, and decay rates. In effect the third-order pattern steers the distribution between the branches while the second-order pattern is active between endings.
• Fourth order = elongation. Elongation as a fourth-order pattern appears to prevent the colliding of branches as long as possible.

The growth pattern is rule-based, but complicated by the fact, that after argon several connection points are growing in parallel. The fractal growth pattern breaks down just after the creation of the 15th backbone icosahedron because the structure folds in on itself and would create physical overlaps if growing further. Once all growth points are used up, element creation comes to its natural end. Therefore, the speculation about the so-called “island of stability” resulting from predictions based on the "shell model," is highly questionable. It is interesting to read the writeup in Wikipedia on this subject which revolves around the notion of magic numbers and double magic numbers of protons and neutrons at Z=114 and N=184. It appears that an enormous amount of effort has been put into this area over the last half century, without much result except deeper and more refined speculation. If the above discussion of fractal growth has merit, that effort would appear to have been futile.